Library
|
|
| Archimedes 3 | |
|
Print Free Pamphlet - - Brief Summary Why 153 Fish in John 21:11 ? Sections :
Introduction Greeks and Wisdom The Greeks excelled in natural wisdom. The even looked to mathematical wisdom as the key on how to live.
Most of the Greeks
probably would not have memorized Archimedes’ method for calculating Pi, but they would have learned about it and how it
used 153. 153 would have been as easily as recognizable to them as 3.14
is to us.
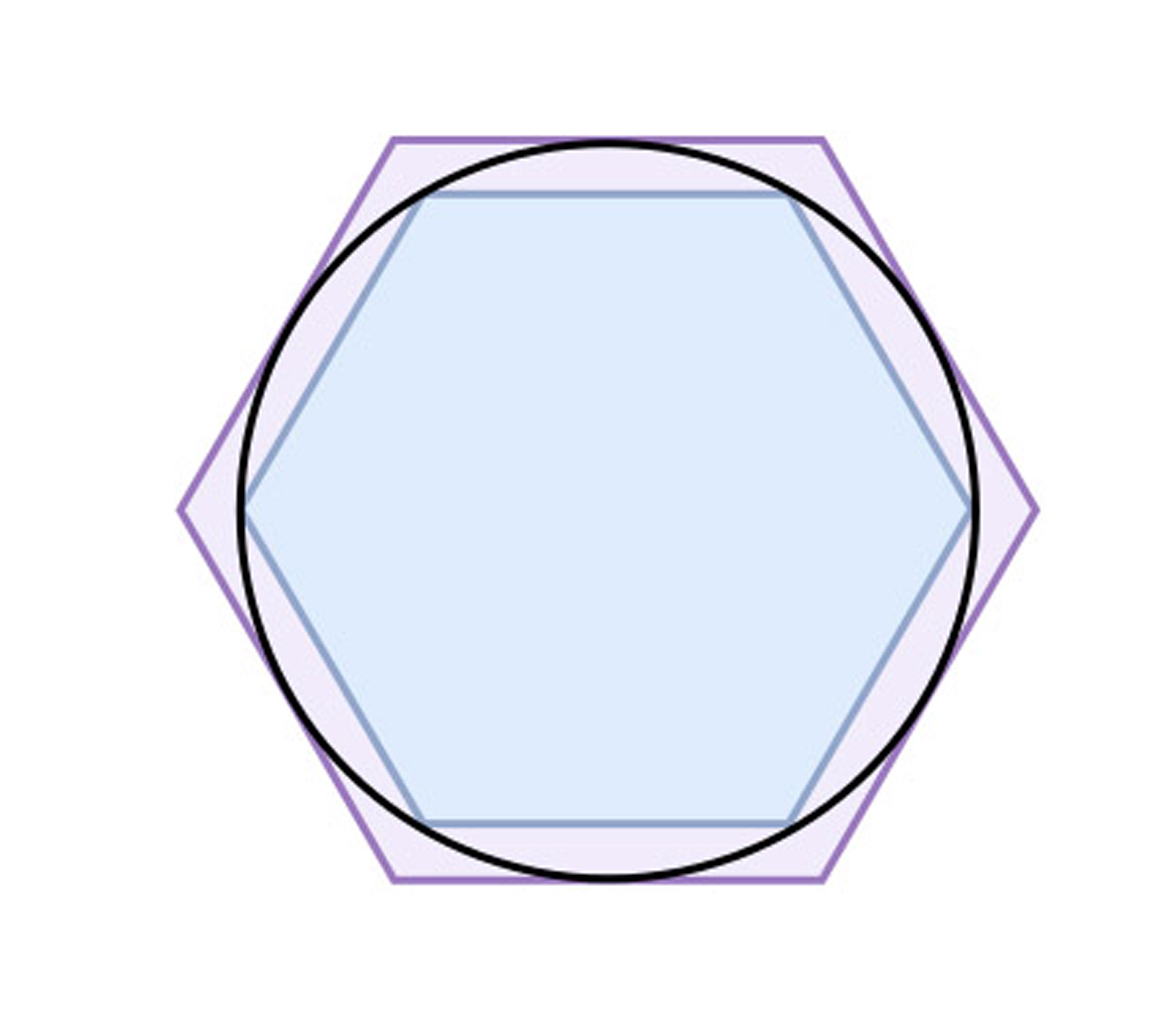
Answer: 1. Archimedes Contribution 1. Archimedes Contribution The reasoning of this objection is based on a misunderstanding what Archimedes accomplished. Civilizations had known about Pi for over a thousand years before Archimedes. 1 Kings 7:23 suggests that Pi is equal, appoxiamately, to 3. One method of determining its value is to measure the circumference of a large circle and to divide it by the measurement of the diameter. However, measurements are inherently inaccurate and subjective. It is difficult to determine the accuracy of a measurement to three or more significant digits. Besides, taking measurements is subjective. How does one prove one measurement is better than another without introducing a third measurement ? Debating which measurement is better is subjective. However, Archimedes new method did not involve taking any measurements. It was pure mathematical calculations. It was completely objective and easily verifiable. There was no subjective argument to dispute. Therefore, the accuracy was easily verifiable. So, it was not so much a particular value of Pi, but rather Archimedes’ method of calculating Pi that was truly remarkable. And so John’s reference to 153 was his way of pointing to this ingenious method that Archimedes developed. Archimedes computed upper and lower bounds of π by drawing a regular hexagon inside and outside of a circle, and successively doubling the number of sides until he reached a 96-sided regular polygon. Using pure math he calculated the perimeters of these polygons in relation to the diameter.
|
|
|
|
|
| This polygonal algorithm was so ground breaking that it dominated for over 1,000 years. It enabled a person to calculate the value of π, Pi, with as much accuracy as one desired. Ludolph van Ceulen, in
1596, in his book Van den Circkel ("On the Circle"), used essentially
the same methods as those employed by
Archimedes
some seventeen hundred years earlier. He calculated Pi to 20 digits, and
later expanded it to 35 digits. So, the important contribution on the part of Archimedes and the Greeks was not so much a particular value for π as it was this the new method of calculating π , Pi. Therefore, it was easier for John to allude to Greek wisdom and to Archimedes’ new method by using 153 than by referring to π itself. 2. No Decimal System Before AD 1100 Also, it is important to note that the decimal system (in America we use the decimal point) with which we use to denote fractions or portions between whole numbers did not come into the west until the 12th century with the introduction of the Indian numerals. So, what Archimedes proved was that Pi was between
Or
Only by using the more modern notation would we be able to write 3.1429 > π > 3.1408 The sequence 314 is a number the Greeks would not have recognized at all. So, the use of 314 was not even an option for John. If John had wanted to refer to Pi he would have been restricted to using one of the numbers that were known and used at the time which was one of the numbers used in the fractional forms of 22/7 or 223/71. The easiest and simplest method for John to allude to this new method for calculating Pi was to use the number “153” as it was much more conspicuous and certainly repeated more often than one of the numbers 7, 22, 71, or 223. This should be seen as obvious when we consider its prominent use in Archimedes’ calculations. As stated above, Archimedes arrived at these values for π by constructing a polygon inside and another outside of a given circle. He constructed these polygons by using 30° - 60° - 90° Δ Triangles. These triangles have unique properties. The ratio of the length of one leg to the other is √3 : 1. This made his calculations much easier. Archimedes used two approximations for the √3 , one greater than its value, and the other less. They are expressed as follows :
|
|
| This equation ends with the number 153. So, by using this number John was able to allude to Archimedes’ work, and therefore to his wisdom.
|
|
|
More Details : See See a more detailed discussion on the importance of the number 153 in
Greek culture. Read :
Continue ... |
|
|
Sections :
Introduction Print Free Pamphlet - - Brief Summary |
|
|
|
|